Can a guess be made more precise? I try with my guesstimate of the Brexit effect
On March 27th I published a post on the Bruegel website (https://moneymatters-monetarypolicy.eu/the-shadow-of-brexit-guessing-the-economic-damage-to-the-uk/) as well as in my blog (https://moneymatters-monetarypolicy.eu/the-shadow-of-brexit-guessing-the-economic-damage-to-the-uk/) that concluded as follows: “the behaviour of growth, investment and inflation in the UK relative to the euro area economy, which is by far its largest trading partner, is consistent with an expectation shock delivered by the result of the Brexit referendum. This has likely caused cumulative lower growth by some 4% while investment would have been some 6% higher. …
In addition, …, Brexit expectations are the weighted average of three very different events: Brexit with no deal, Brexit with a deal, and no Brexit. There is no objective measure either of the probabilities to be attached to the three events or of their economic consequences. Audacity is needed to build on such frail basis. Still, it may be illustrative to carry out some quantitative, yet arbitrary, exercise to extrapolate, from what has happened so far, the possible consequences of a revelation of one or other of the Brexit outcomes.
Let’s start from the estimated effects on growth and investment, via expectations, of the negative result of the Brexit referendum: four and six percentage points respectively for growth and investment. Let’s call these estimates generically Z. Let’s further call the probabilities of the three possible events, no-deal Brexit, deal-Brexit and no Brexit P1, P2 and P3, while x1, x2 and x3 are the damages expected from the three events. With these symbols, we can write:
x1P1 + x2P2 + x3P3 = Z
where it is assumed that, through expectations, the damage expected in the three events, weighted by the relative probabilities, have been anticipated, resulting in the effect Z, even before Brexit.
By inserting some heroic assumptions into the equation, we can derive what the effect would be if one or the other event finally materialised. In a way the exercise is to scale up, or down, Z depending on whether x1, x2 or x3 eventually prevails.
The first, most innocent but not totally innocent, assumption is that x3 = 0, i.e. there are no negative consequences if eventually there is no Brexit. Then one needs assumptions on P1 and P2 as well as the damage from Brexit without a deal, relative to the damage of Brexit with a deal, i.e. x1/x2= k. With these assumptions, one can calculate x1 and x2, i.e. the consequences of Brexit without or with a deal.”
In the post I assumed, faute de mieux, that P1 = P2 = 0.33 and x1/x2 = k = 2. Now I have read in the Economist issued on March 30th that Brits have been betting on the Brexit outcome and a probability of no deal was extracted from these bets for the period January 15th to March 20th, ranging between 0 and 25 %.
On this basis I can make my guess more precise and review the chart I had inserted in my previous post, reproduced here as Chart 1, reporting the damage resulting from no-deal Brexit and deal-Brexit along different probabilities of no-deal (P1) as incorporated into expectations (in the horizontal axis), with the following assumptions:
- The probability of no Brexit is 0.33
- The damage from no-deal Brexit is the double of the damage from a deal-Brexit (k=2).
Chart 1. Damage resulting from no-deal Brexit and deal-Brexit.
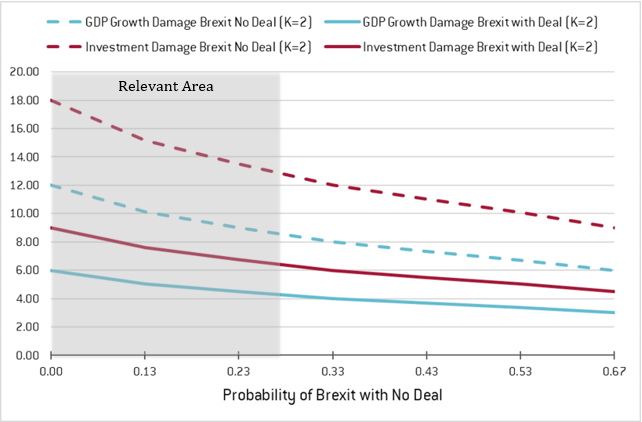
Author calculations.
Given the bet-derived probability of no deal, the relevant part of the chart is the shaded one on its left, where the estimated damage, for both investment and growth, is highest. This is because the anticipated damage, Z, has taken place even if expectations were optimistic (little probability of no-deal Brexit is built into them). This must mean that the expected damage from Brexit is large. The opposite happens in the right part of the chart in which pessimistic expectations are reported (high probability of no-deal Brexit is built into the expectations) and the damage actually produced is still Z.
In other words, given the little fear that there would be no-deal Brexit, as revealed by the bets, damage Z must have been generated by the expectation of large negative effect from Brexit (high values of x1 and x2). For instance, looking at the bet-derived range of expectations of no-deal Brexit, the zero probability would mean that the damage that would generated if this event, contrary to expectations, eventually prevailed would be around 12% for growth and 18% for investment; if the probability of no-deal Brexit was 25%, the damage from this event would be around 9% for GDP and 13% for investment.
The bottom line is that the future damage from Brexit, in particular in the case of no deal, will be much larger than the relatively limited damage that the expectation of Brexit has already caused.
Any reader as audacious as me, wanting to use her/his assumptions about the probabilities built into expectations as well as the relative damage of deal-Brexit vs. no-deal Brexit, could introduce them into the little spread-sheet enclosed with this post to see which results would be generated. Audacity should not be pushed, however to the point of taking the generated results as a true forecast of what could happen if any of the three Brexit variants would indeed materialise.